In the previous article I introduced synthetic scales, which made us dig up an element of scales and modes which we had already touched upon before: tetrachords. So before we move on I think it's important to highlight the interest of splitting scales and modes into tetrachords and then put them back together.

Splitting and combining scales and modes
This splitting and re-combining is interesting in three ways. First of all, it allows you to consider scales and modes beyond their entirety and look at the elements, or “bricks” if you prefer, that constitute them. And since these “bricks” are less numerous than the scales and modes they help build, they are easier for any musician to eventually learn, while at the same time providing a new understanding of what he/she already knows. The easier it is for the brain to connect things, the better it can master them.
The second advantage is being able to use simple tools to create new structures, the famous synthetic scales. And, finally, the third advantage is directly related to the previous one: synthetic or hybrid scales allow you to create coherent melodies even within harmonically rich and changing environments.
After this brief introduction, how about we start putting some scales together?
Synthetic scales from tetrachords of natural modes
In articles 49 and 64, we focused on the tetrachords of natural modes, in other words, the modes of the natural major scale (including the natural minor scale, the Aeolian mode or the sixth mode of the major scale). If you combine them, in addition to the modes in article 49, you get the following results:
Putting together a minor and major tetrachord, you get a melodic minor scale:

Combining a major tetrachord with a Phrygian tetrachord you get a Mixolydian b6 mode (5th mode of the melodic minor scale):

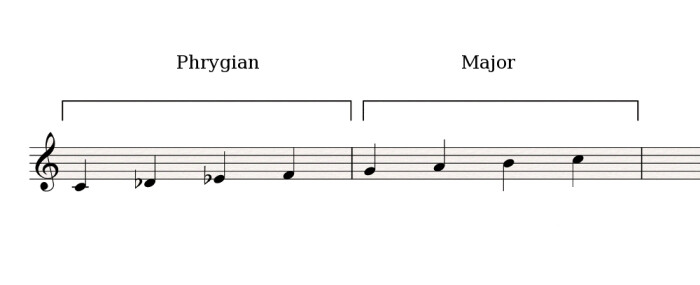
Mixing a Phrygian tetrachord and a major tetrachord you get a major Neapolitan mode:

Combining a Phrygian tetrachord and a minor tetrachord you get a Phrygian natural 6 mode (2nd mode of the melodic minor scale):

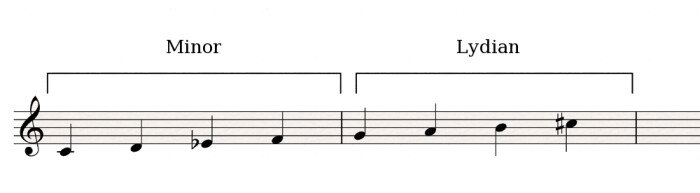
Putting together a Lydian tetrachord and a minor tetrachord you get a Lydian b7 mode (4th mode of the melodic minor scale):

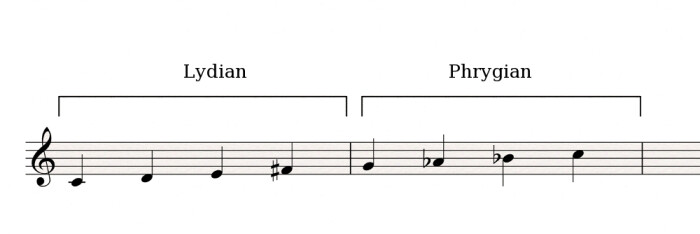
Combining a Lydian tetrachord and a Phrygian tetrachord you get the third mode of the whole tone scale (well talk about this scale more in detail later on):

Synthetic non-octaving scales from tetrachords of natural modes
The following combinations result in the so-called “non-octaving” scale, because they do not allow the repetition of an octave structure. We will also talk about them in a future article.
Combination of a minor tetrachord and a Lydian tetrachord:

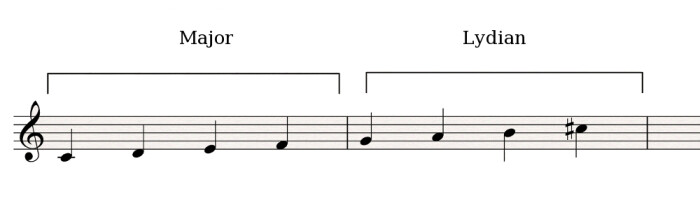
Combination of a major tetrachord and a Lydian tetrachord:

Doubling of a Lydian tetrachord: