In the previous article you learned that Lydian tetrachords were absent from the modes of the melodic minor scale. But since we really like the oddest combinations, I suggest we wrap up this brief review of the different possibilities offered by the use of tetrachords looking at what happens when you combine Lydian tetrachords with the new tetrachords of the melodic minor scale.

Synthetic scales of the harmonic minor scale modes and the Lydian tetrachord
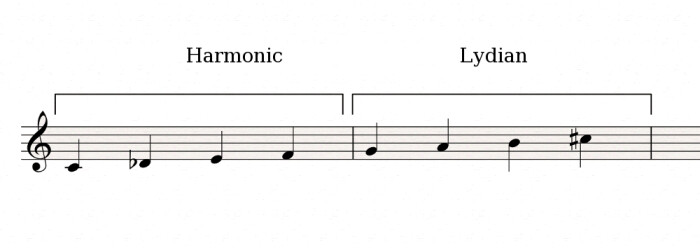
Since the Lydian tetrachord is missing from the modes of the melodic minor scale, I invite you to discover the results of putting it together with the new specific tetrachords of these modes.
As you’ve seen in previous articles, the presence of one or even two augmented tetrachords essentially results in the formation of scales which go beyond an octave.
Combining a Lydian and a harmonic tetrachord you get:

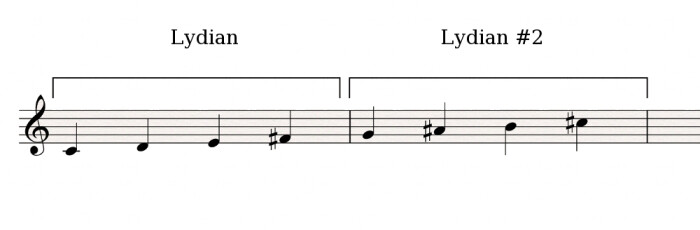
Combining a Lydian and a Lydian #2 tetrachord you get:

Combining a Lydian and a minor #4 tetrachord you get:

Combining a harmonic and a Lydian tetrachord you get:
Combining a Lydian #2 with a Lydian tetrachord you get:

Combining a minor #4 and a Lydian tetrachord you get:

It’s easy to see that the separation between the two tetrachords of all non-octaving scales created is a whole tone. But what happens when instead of separating the new synthetic scales with a whole tone you do it with a semitone, lowering the second tetrachord a semitone?
Synthetic scales of the harmonic minor scale modes and the Lydian tetrachord… separated by a semitone
Well, you get the following:
Putting together a Lydian and a Lydian #2 tetrachord separated by a semitone you still get a non-octaving scale:
Putting together a Lydian and a minor #4 tetrachord separated by a semitone you still get a non-octaving scale:

Putting together a Lydian #2 and a Lydian tetrachord separated by a semitone you still get a non-octaving scale:
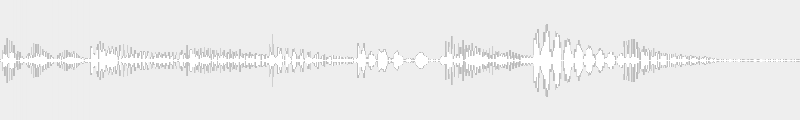
Putting together a minor #4 and a Lydian tetrachord separated by a semitone you still get a non-octaving scale:

However, combining a Lydian and a harmonic tetrachord separated by a semitone you get a “real” harmonic Lydian scale:
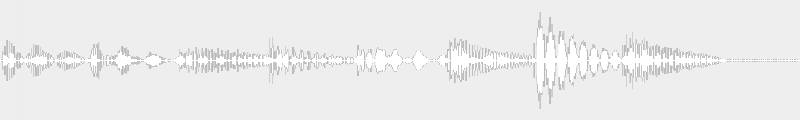
And putting together a harmonic and a Lydian tetrachord separated by a semitone you get a so-called Asian scale:

As you can see, some synthetic scales are non-octaving when their tetrachords are separated by a whole tone but they become octaving when those same tetrachords are separated by a semitone instead. This obviously is in detriment of the original harmonic content, so it’s up to you to combine the tetrachords the way you see fit. Try it yourself with the non-octaving scales created in the previous articles.
In the case where the tetrachords of the synthetic scales are only separated by a semitone, some notes acquire a new status as leading-tones. But that’s something we’ll discuss in the next article: creating synthetic scales by making leading tones out of the notes of pre-existing modes.