If you think of music as a language, then you could say that it's made up of phrases, with cadences acting like punctuation marks.
In this article we’ll go back and look at the perfect cadence we previously mentioned and study the other main cadences there are.
The perfect cadence
As you have seen in the previous articles, not only does each degree of a scale has a specific function, but the 5th degree followed by the 1st degree results in the so-called perfect (or authentic) cadence, which often serves to end a song, or at least a musical phrase. [Ed. note: A cadence is a chord progression, usually at the end of a phrase, which signifies closure of some sort.]
I’ll make a brief parentheses here to illustrate the historical importance of these degrees in a scale. Between the 5th and 6th century of this era, the philosopher Boëce advocated for the use of simple 2:1 or 3:2 mathematical ratios in architecture and music. Back then, music was considered as a science modeling the world. It was believed that it could even affect human health, both positively and negatively. So, the 2:1 and 3:2 ratios correspond to the ratios between the root and its first harmonics, the octave (2:1) and the fifth (3:2), in other words, the I and V degrees (to learn more about harmonics, refer to part 3 of the Sound Synthesis, Sound Design and Audio Processing series).
Since the perfect cadence is often used to end a musical phrase or an entire song, as I mentioned before, you could say it acts as a sort of period in written language. As its name implies, the perfect cadence represents the most important form of cadence.
When it comes to the perfect cadence, it’s often said that the dominant chord makes an approach to try to reach the scale’s root note. To reinforce this effect, the four-note seventh dominant chord is used instead of the triad (see part 4). In a future article I’ll explain why this is so.
To get used to chord inversions, the following examples are all made up of a melodic line in the upper part, harmonized with second inversion chords, except for the final chord of each phrase, which is in its root position. The only cadence form that contravenes this “rule, ” to preserve its characteristic sound signature, is the chord progression known as “rhythm changes.” I’ll let you discover by yourself which chord inversions I used!
Let’s see now an example of a major perfect cadence:
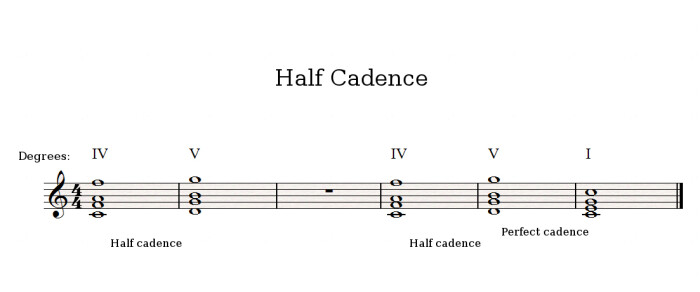
The half cadence
A particular form of the perfect cadence is the half cadence (aka imperfect cadence or semicadence). In this case it’s not a period, but more like a comma because it doesn’t mark the end of a phrase, but rather revitalizes the discourse. It’s also called “dominant cadence” because it doesn’t lead to the root, but rather… to the dominant. It’s often found preceding a perfect cadence.
In the next example, you will first hear the half cadence on its own an then the half cadence followed by the perfect cadence. Do note that, when it comes to the half cadence, the degree that leads to the V isn’t necessarily the IV. I used it in this example to try to keep some coherence regarding all the examples in the article.

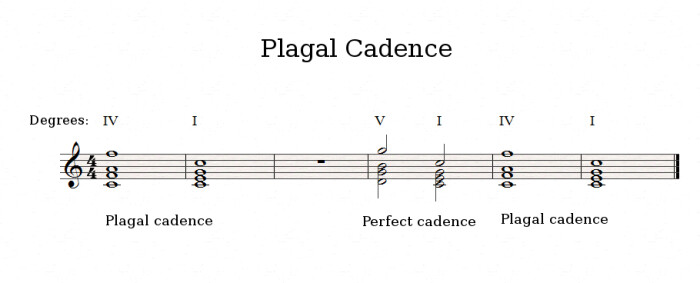
The plagal cadence
Next is the so-called plagal cadence, defined by a progression from the IV to the I degree of the scale. It doesn’t have the same force as the perfect cadence and is only rarely used to finish a song. However, it can be useful to emphasize even more the final perfect cadence of a song.

Rhythm Changes
In harmony, rhythm changes refer to one of the most famous cadence forms. It’s basically a progression of the VI, II, V, and I degrees in this exact order or in the I-VI-II-V form (followed, more often than not, by the I degree).
Also note that it can be considered as a sort of chained perfect fifth drop to the lower fifth, starting from the VI of the song’s key and ending on the root note.
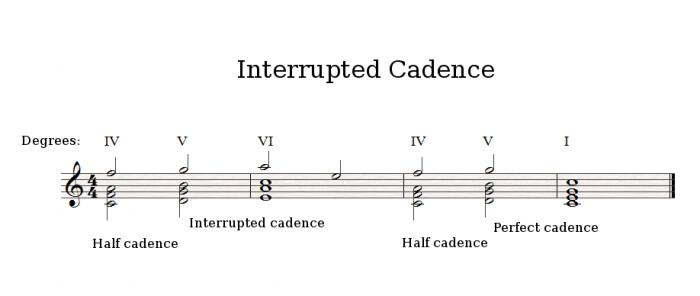
The interrupted cadence
Finally, there’s the so-called “interrupted” or “deceptive” cadence, which differs from the perfect cadence in the sense that it does not end on the I but on the VI degree. This means you have a V-VI progression. So, instead of finishing a song, it allows you to give it a different harmonic color, a trick often used before actually finishing the song. And in the case of a major scale, this other harmonic color is none other than…the relative minor of the song, since the VI degree of the scale corresponds to the relative minor of this same scale!

In the previous example, by resolving to the VI degree of the key you could actually shift the latter to its relative minor, A minor in this case. This is a typical example of “modulation, ” which basically means you use a degree of a given scale to move to another key. Yes, we’ll look into modulations in an upcoming article.
Next week, to continue our quest for the perfect harmonization, I’ll show you how to determine which notes belong to which chords and keys, by studying “chord” and “none-chord” tones.