In the previous article, we explored sounds based on waveforms with more or less harmonics, but they all had one thing in common: They were periodic. As we saw, if you refer to periodic cycle, you refer to frequency, and if you refer to frequency, you refer to pitch and...voilà, you have a music note. Today, we'll deal with sounds that aren't periodic, in other words, noise.
I can already hear detractors of electronic music having a field day: “I always said electronic music was nothing but noise!” OK, that was an easy joke, I’m sorry…
Anyway, noise is a fascinating sound material and there are different types of noise. In fact, when it comes to sound synthesis, we have normalized and labeled them according to a very suiting color code.
White Noise
White noise gets its name from white light ─ which, as you know, is the sum of all existing colors ─ and represents the sum of all existing frequencies with constant power across the entire frequency spectrum. But that’s only the theory. In fact, it only includes frequencies within certain limits, like those of human hearing (20Hz –20,000Hz, in case you forgot). If white noise had an infinite number of frequencies, it would have a constantly infinite power ─ God complex, anyone?
If you recall the definitions on previous articles, white noise is the perfect example of a complex aperiodic sound.
From a practical point of view, it’s the sound emitted by a TV when there’s no program on any given channel.
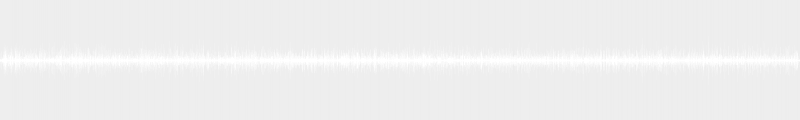
Pink Noise
Pink noise, which isn’t as common as white noise on synthesizers, is specially used in audio applications to test or calibrate sound systems. White noise is unfit for this task for the following reason:
If you recall the table of frequencies in our “Sound synthesis, sound design and audio processing – Part 3” article, you can easily see that the frequency range covered by the last octave is much more significant than the one covered by the first one. The sound energy of white noise – all frequencies at a constant power ─ is much more considerable in the higher than in the lower octaves. With such a lack of balance it’s impossible to calibrate anything at all!
Pink noise is a white noise whose sound power decreases 3dB per octave (or with every frequency doubling), in order to get a homogeneous power across all octaves.
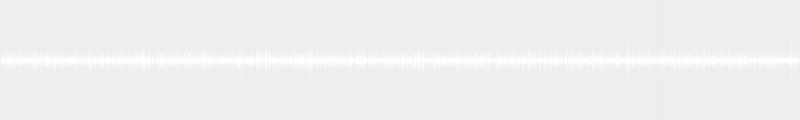
Brownian (or Red) Noise
To understand where this type of noise comes from we will need to take a look into botanics and thermodynamics ─ easy as that!
In 1827, while looking through a microscope, Scottish botanist Robert Brown discovered that pollen particles in water experience continuous but irregular disturbances. This phenomenon was later called “Brownian motion” after its finder. But it wasn’t until the early 20th century when Einstein and ─ independently ─ Smoluchovski proposed a valid theory explaining the phenomenon: The motion of these pollen particles resulted from them bumping into the particles of the water surrounding them. This theory allows, among other things, to explain the relationship between the pressure, temperature and volume of gas.
But aren’t we deviating from music? Well, not really, since Browninan motion can also be applied to a complex aperiodic waveform.
By listening to these audio examples, you’ll notice that each of the noises seems to be busier in the lower frequencies than the previous one. Which is pretty normal: As we just saw, pink noise frequencies drop 3dB every octave, while the SPL of brownian noise frequencies decreases 6dB per octave.
Other Noises
There are also other types of noises (blue, violet, gray), but you will hardly ever find them in sound synthesis, so there’s no need go into any details about them here.
And there seems to be yet another type of noise, called “brown noise” (not to be confused with the Brownian noise, also incorrectly called “brown noise” sometimes) which, according to the makers of South Park, consists in a frequency range that produces an uncontrollable urge to go to the toilet… Up to now, however, there is no scientific evidence that corroborates this interesting theory.
